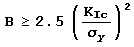
Eq. Te-1 [1]. The minimum thickness of material before plane strain behavior occurs.
Overview:
One of the most important properties of any material for virtually
all design applications is fracture toughness. Given the unusual
units of MPa(m1/2), fracture toughness is a quantitative
way of expressing "a material's resistance to brittle fracture
when a crack is present." [5]. If a material has
a large value of fracture toughness it will probably undergo ductile
fracture. Brittle fracture is very characteristic of materials
with a low fracture toughness value.
Differences between Fracture Toughness:
There are actually four different types of fracture toughness, KC, KIC,
KIIC, and KIIIC.
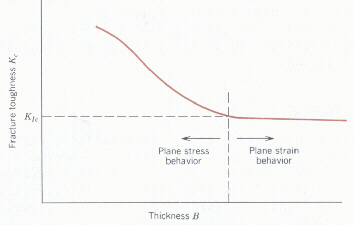
KC
is used to measure a material's fracture toughness
in a sample that has a thickness that is less than some critical
value, B. When the material's thickness is less than B, and stress
is applied, the material is in a state called
plane stress.
The
value of B is given in equation Te-1. A material's thickness is
related to its fracture toughness graphically in figure Tf-1.
Equation Te-2 shows a material's KC value in relation
to the material's width.
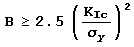

Eq. Te-2 [1]. The fracture toughness of a material with a thickness less than B.
KIC,
KIIC, and KIIIC all represent
a material's fracture toughness when a sample of material has
a thickness greater than B. If a stress is applied to a sample
with a thickness greater than B, it is in a state called plane
strain. The differences between KIC, KIIC,
and KIIIC, however, do not depend on the thickness
of the material. Instead, KIC, KIIC, and
KIIIC are the fracture toughness of a material under
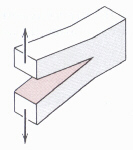
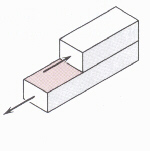
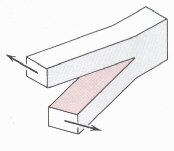
the three different modes of fracture, mode I, mode II, and mode
III, respectively. The different modes of fracture I, II, and
III are all graphically expressed in figures Tf-2, Tf-3, and Tf-4.
Equation, Te-3 shows how KIC can be calculated knowing
the material's parameters.
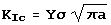
KIC values can be used to help determine critical
lengths given an applied stress; or a critical stress values can
be calculated given a crack length already in the material with
equations Te-4 and Te-5.
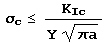
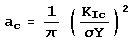
Callister provides a table, Table Tt-1, of fracture toughness
of common engineering materials.
Students may notice that the ceramic materials have a much lower
KIC value than the metals. The low KIC
value reflects the fact that ceramic materials are very susceptible
to cracks and undergo
brittle fracture, whereas the metals undergo
ductile fracture.
K1C values are determined experimentally. If you
would like to learn about how these experiments are conducted,
click here on this link.
Example Problem : Fracture Toughness
Plates of a ceramic material called boron carbide are being used
to absorb neutrons in a nuclear reactor. Boron carbide has a
KIC of approximately 4 MPa(m)1/2 and a high
yield strength of 400 MPa. The compressive stresses being applied
to the boron carbide plates, only 10-3 m thin, are
about 5 MPa; Y = 1.1. What is the critical crack length in the
boron carbide plates?
Answer:
Step 1) Are the ceramic plates thick enough to be in plain strain
when a stress is applied to them?
B = 2.5 (KIC / sy)2
B = 2.5 (4 MPa(m)1/2 / 400 MPa)2
B = 2.5*10-4 m
The width of the material is greater than 2.5*10-4 m, therefore plane strain
applies to the problem
Step 2) Plug the known and calculated values into the equation
given and calculate the critical crack length.
ac = (1 / Pi) (4 MPa(m)1/2 /
(5 MPa * 1.1)2
ac = 0.168 m
Submitted by Matt Gordon
Virginia Tech Materials Science and Engineering
http://www.eng.vt.edu/eng/materials/classes/MSE2094_NoteBook/97ClassProj/exper/gordon/www/gordon.html
Last updated: 4/25/97