Strain Invariants and Dislocation Core Structure of Simulated B2 NiAl

by J. Kevin Ternes

Abstract

This project established a methodology and infrastructure for the generation of images and movies of dislocation cores showing the deformed crystal lattices and their associated strain invariant fields. Many examples of these images as well as several movies generated from these images are presented in the following discussion. These images show a B2 crystal lattice of NiAl (shown below) which is deformed due to the presence of various dislocations superimposed over the appropriate strain invariant fields, Y1 and/or Y2, whose magnitude has been mapped to color or plotted as contours. The movies were made by assembling sequences of images generated from the simulation data where a simulation parameter has been varied slightly from frame to frame so that the parameter is, in effect, cast into time.

Data for these images was generated via atomistic computer simulation using embedded atom potentials. For this project, computations are carried out simulating a variety of dislocations in the B2 phase of NiAl although it is possible to apply these visualization techniques to the results of most any intermetallic alloy simulation. Calculations were done for both stoichiometric and non-stoichiometric simulation blocks with varying degrees of applied shear.

Simulation Results

Nickel--Aluminum

Relaxed lattice of the stoichiometric pure edge dislocation, l=[0-10],[100](001).

A stoichiometric pure edge dislocation l=[0-10], [100](001) with varying shear was simulated. The Peierls stress was found to be 2600MPa for the lower energy Ni-rich core and 3700MPa for the Al-rich core. The relaxed lattice along with the resulting Y2 invariant field of the lower energy configuration may be seen above. Ni atoms are blue while Al atoms are pink. The same dislocation may be viewed as an MPEG movie where increasing applied shear has been cast over time such that changes in the core's structure and Y2 strain field may be clearly seen before during and after the Peierls stress is reached. The shear has been applied such that glide to the left will occur. The animation shows a great deal of spreading in the strain field in the direction in which slip will occur. Note that the strain that has built up is periodically released as movement occurs and although the strain field is changing as stress is applied, the lattice itself does not appear to change unless movement is occurring.

The same pure edge dislocation was simulated in Ni-48Al by randomly substituting Al atoms with Ni atoms for different random seeds. Peierls stresses of 4000MPa and 3000MPa, respectively, were found for the Ni- and Al-rich cores. The relaxed and unstressed Ni-rich cores resulting from two different random seeds may be seen for:

seed1, seed2, and seed4.

The Ni-rich core was again simulated, this time with singly placed antisites and vacancies. Images of Ni antisites, Al vacancies and Ni vacancies (which are thought to be energetically unfavored) were generated. Note that in the images below, the Ni vacancy causes no visible change in the core structure and very little change in the strain field even though it was placed almost at the center of the core:

Ni antisite 1 , 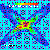Al vacancy 1 and Ni vacancy 2 .

Multiple Al vacancies were placed at key positions with respect to the core in the simulations resulting in the following images:

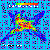vacancy configuration 3 and 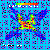vacancy configuration 4 .

Other dislocation geometries were simulated on the same slip plane having a mixed screw and edge character. Such dislocations have a Y2 as well as a Y1 component to the strain invariant. Images and Peierls stresses are:

Numerous simulations were carried out on the (01-1) slip plane. The l=[011], [100](011) pure edge dislocation was simulated for various stoichiometries and the Peierls stresses were found. The Ni-48Al simulations were found to be not sensitive to placement of the antisites whereas the Ni-42Al simulations were found to be very sensitive to the given random seed:

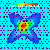Ni-50Al, 410MPa , Ni-48Al, 520MPa and Ni-42Al, 250MPa .

The MPEG animation of this pure edge dislocation , indicates that the outer limbs of the invariant field slowly contract before the core moves. The onset of movement is quite sudden and occurs at an applied shear of 410MPa. Other than the contraction of the strain field, there is no warning that glide is about to occur. As the core moves, observe the deformation in both the lattice and in the strain field which is represented as color. This dislocation has also been simulated with individual Al vacancies introduced in the core region. Images are given for Al vacancies in:

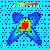position 1 , position 3 , position 5 and position 8 .

Two pure screw dislocations were simulated. The first, l=[001], [001](010), exhibited notable behaivior under shear. Although the shear was applied such that the dislocation would glide in the (010) plane, the dislocation moved instead along the (011) plane at a Peierls stress of 100MPa.

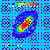The pure screw dislocations, l=[001], [001](010) and l=[100], [100](01-1).

The second pure screw, l=[100], [100](01-1), was found to have a Peierls stress of 390MPa (shown) or 70MPa depending upon its initial configuration as discussed below. The MPEG animation of this pure screw dislocation shows the Y1 strain field undergoing a very slow contraction before sudden changes which occur at both 316MPa and 390MPa. In two steps, the core appears to reorient its longer axis along the direction of applied stress and then glide easily. Notice that, except for the orientation, the core appears unchanged before and after this transformation.

In fact, the two orientations of the screw are crystallographically equivalent. We have found that this core glides easily along its longer axis at 110MPa if the shear is initially applied along that axis. Even though the two core configurations are energetically equivalent, an energy barrier must be overcome in order to change between the two. This barrier accounts for the difference in the Peierls stresses.

Nickel--Aluminum--Titanium

More recently, we have simulated B2 NiAl with titanium impurities. It is thought from just energy considerations that the Ti impurities will prefer to substitute themselves into the Al sublattice. Here are such simulations of Ni-47Al-3Ti and Ni-44Al-6Ti for the l=[0-10], [100](001) dislocation discussed above. The Ti atoms are represented in green. For comparison, simulations where the Ti atoms were substituted into the Ni sublattice were carried out. Here are images of 47Ni-50Al-3Ti and 44Ni-50Al-6Ti for the same dislocation where much higher strains have manifest illustrating our notions of that such substitutions are disfavored.

Information about how these data and images were generated.

Figure: The B2 crystal structure.

This work was done by Kevin Ternes as a project in the class "Scientific Visualization and Multimedia" and was used in partial fulfillment of the requirements for a Master of Science Degree in Materials Science and Engineering at Virginia Tech which he received in December of 1994. His thesis is entitled, "Visualization and Simulation of Defect Structure in the B2 Phase of NiAl"
Strain Invariants and Dislocation Core Structure of Simulated B2 NiAl / VPI&SU / jkternes@interramp.com

Click here to go to the 1994 Scientific Visualization and Multimedia class page.